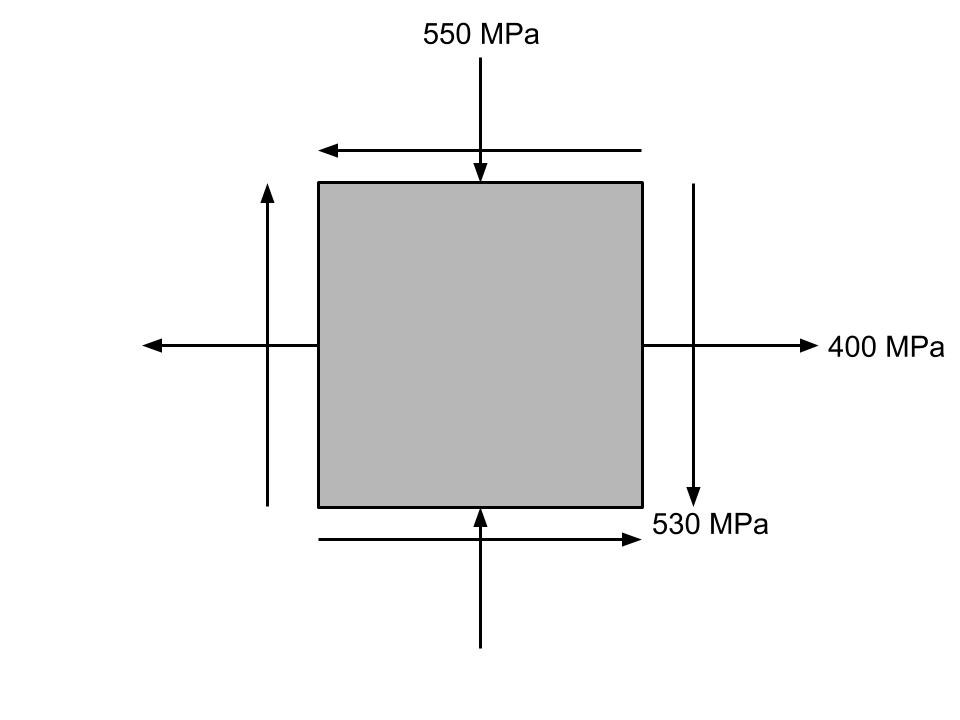
FE Exam Practice Question: What is the angle of rotation needed to get the stress element in the maximum principal stress state?

This can come in several NCEES FE Exams: Civil, Mechanical, and Other Disciplines.

Solution:

This is a common problem you can encounter on the FE exam, the PE exam, and in school. For the FE exams, you'll find it in these topics:

1. FE Civil - Mechanics of Materials - Combined stresses, principal stresses, and Mohr’s circle

2. FE Mechanical - Mechanics of Materials - Stress transformations and Mohr's circle

3. FE Other Disciplines - Strength of Materials - Stress transformation and principal stresses, including stress-based yielding and fracture criteria (e.g., Mohr's circle, maximum normal stress, Tresca, von Mises)

---

Ultimately, we must determine the rotation angle needed to get the stress element (stress block) to a principal stress state.

Steps to determine the angle of rotation needed:
1. Draw a quick Mohr's circle based on what's shown in the stress element.
2. Draw a right triangle from the center of the Mohr's circle to a known point along the circle. Determine the Mohr's circle's angle between the known point and the sigma axis (x-axis), which is where the principal stress occurs. That will be the Mohr's circle rotation angle needed to get to a principal stress state.
3. Convert the Mohr's circle's rotation angle needed to get to a principal stress state to the stress element's angle needed to get to a principal stress state.

Step 1. Draw a quick Mohr's circle based on what's shown in the stress element.

Normal stresses (σ_x, σ_y) in tension (going away from the stress element) are positive and normal stresses in compression (going into the stress element) are negative. For shear stresses (τ_xy) look at the right side of the stress element. If the right shear arrow pulls the stress element clockwise, then the shear is negative. If the right shear arrow pulls the stress element counter clockwise, then the shear is positive. Therefore,

τ_xy = -530 MPa
σ_x = 400 MPa
σ_y = -550 MPa

Calculate the center of the Mohr's circle.

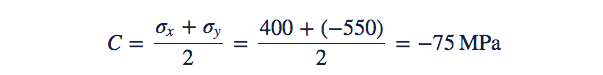
With the known info, plot a random point along the perimeter of the circle.

(σ_x,τ_xy) = (400,-530)

Step 2. Plot a right triangle from the center of the Mohr's circle to the known point along the perimeter of the circle.

Use Pythagoras Theorem to solve for the hypotenuse of the triangle, which is also the radius of the circle.

a²+b² = hypotenuse²

(530 MPa)²+(75+400 MPa)² = hypotenuse²

hypotenuse = radius = 711.7 MPa

Calculate the Mohr's circle's angle to a principal stress state. Use soh-cah-toa.

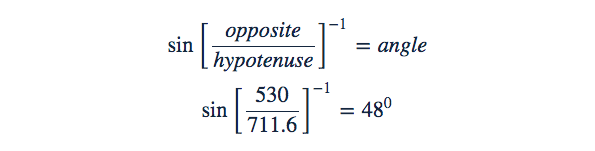
Step 3. Convert the Mohr's circle's rotation angle needed to get to a principal stress state to the stress element's angle needed to get to a principal stress state.

The angle, 2θ, is the Mohr's circle's angle to get to a principal state, it is NOT the stress element's angle to get to a principal state. This problem asks for the angle to get the stress element to a principal state. Therefore,

Mohr's Circle's angle = 2θ = 48°

Stress element's rotation angle to a principal state is θ, therefore 48/2 = 24° clockwise rotation.